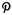Think Like a Disciplinarian

I have been working VERY hard to recreate the black and white think like a disciplinarian (TLAD) pictures our GT trainer gave us.  I've been searching TpT for the perfect clip art and I finally have a set that I am very happy with.  I want to give a HUGE thank you to Ron Leishman at Digital Toonage for helping me!  He's amazing.  I sent him a message asking for some specific fields like entrepreneur, statistician, historian and physicist and he did an AMAZING job!  I highly recommend his work to anyone and he's super accommodating! These poster have two versions- one with a  small paragraph explaining the field and one without.  Print them on cardstock and laminate and you will be able to use them in so many different ways.  Teaching just math last year, I was not able to use them as much as I wanted to but each time we did a PBL, I was able to incorporate disciplinarian work. For example, in the fall when we did a project about designing a fall festival students learned about entrepreneurs, graphic designers, sociologists and environmentalists. Throughout the project, each of those disciplinarians had specific things that were relevant to them. The entrepreneur was concerned about choosing booths that made the most profit. The environmentalist was concerned about booths that adversely affected the environment. The graphic designer was concerned about making the best logo and brochure. And the sociologist was interested in setting up the festival so there was enough time and space for human interactions. Another project I did at the end of the year- the classroom makeover- also incorporated TLAD. I will describe that more in an additional post.
My ELA teammate used disciplinarians when reading novels with students. She had students thinking like a veterinarian while reading Because of Winn Dixie and thinking like an engineer when reading City of Ember. There are SO many uses for thinking like a disciplinarian!  Here are a few samples from my product. Here's a link to the product here

Scholarly Behaviors

I have a new job- I am the gifted specialist for a school in my district. I am beyond excited to begin this new journey. In preparation for my new job, I am taking another look at my Scholarly Behavior posters I made last year. I added some captions for each poster and finally uploaded them to TpT. You can find them here. These scholarly behaviors are based on the work of Dr. Sandra Kaplan. These are eleven different traits/skills that scholars should have or be able to do:

• Academic humility
• Intellectual risk-taking
• Academic Preparation
• Ponder Ideas
• Intellectual curiosity
• Save and organize ideas
• Exercise Intellect
• Goal-setting
• Perseverance in learning
• Use many and varied resources
• Multiple perspectives

Last year I used them in my 4th grade math classroom a lot. At the beginning of the year, I introduced the behaviors from the very beginning. The first day of school I asked my students to complete a sheet that looked like this:

I asked students to think about the math with which they already feel confident. I wanted them to write those concepts in the glass. Then around the side I asked student to write down things they want to learn this year in math- expressing their intellectual curiosity.

We were departmentalized this past year in 4th grade and it was important that student knew what materials they needed for each class. We created this organizer to help them the first week. In each class, students wrote down the subject and what materials were needed for that specific class.

Another way I introduced the behaviors was by graphing pre-test data and talking about the behavior of goal-setting.

I also used Save and Organize Ideas when we set up interactive notebooks.

Throughout the year we referred to these scholarly behaviors a lot in all subject areas. My language arts teammate would refer to these behaviors when discussing how characters acted in certain stories or how famous people reacted to events in their lives when reading biographies. In all our classrooms, these posters hung in prominent locations and students referred to them often. Here are some sample pictures from the product.

The set comes with four different choices- 2 font styles and then within both font styles there are posters with a caption and without a caption.

Chocolate Hearts

Act 1:

Chocolate Hearts from karla heleotes on Vimeo.

What do you notice?
What do you wonder?


Main Question: How many chocolates are left?


What's an estimate that is too high?
What's an estimate that is too low?
What's a reasonable estimate?

Act 2:

What information do we need to solve this?

The total number of chocolates

The number of each type of chocolate that was eaten

Act 3:

What strategies did you use to solve this?

How close was the answer to your estimate?

13 chocolates are left.

The Heart Jar: A Valetine 3-Act Task

Act 1

The Heart Jar Act 1 from karla heleotes on Vimeo.

What do you notice?
What do you wonder?

How many hearts fit into the jar?

What's an estimate that is too high?
What's an estimate that is too low?
What's a reasonable estimate?

Act 2
What information do you need to solve this?

These are the number of candies in one box.

Six full boxes were used.

Some candies from the seventh box were used. These are the leftovers.

Act 3
What strategies did you use to solve this problem?  How close was the answer to your estimate? 

My First 3-Act Task

I've loved going into classrooms and modeling 3-act tasks. Graham Fletcher has some amazing 3-act tasks on his website. https://gfletchy.com/3-act-lessons/

But I felt like it was time to try my hand at it and make a few of my own.  Since Valentine's Day is coming up soon, I decided that this was the perfect theme for the 3-act tasks I would create.

Disclaimer: Please be kind- these as my first attempts...

Sharing Hearts
Act 1
Watch the video.

Sharing Hearts from karla heleotes on Vimeo.
What do you notice? What do you wonder?

If they share evenly, how many hearts will each student receive?
What's an estimate that is too high?  What's an estimate that's too low?  What would be a reasonable estimate?

Act 2

There are 2 girls sharing.

Act 3

What strategies did you use to solve this?


Sharing Hearts from karla heleotes on Vimeo.


How close was your estimate to the actual answer?

The Power of Mistakes in Math

I am reading this amazing book by Jo Boaler called Mathematical Mindsets.
In it she references Carol Dweck's research on growth mindset and takes it a step further to make some needed connections to math teaching and learning in the United States and Great Britain. She discusses some work by psychologist Jason Moser. He studied the neural mechanisms that operate in people's brains when they make mistakes. He stated that there are two possible responses in the brain when we make a mistake. The first is called an ERN response which is an increased electrical activity when the brain has conflict between a correct response and an error. This brain activity occurs whether or not a person knows they have made a mistake. The second response, called a Pe, is a brain signal when we know we've made a mistake. It happens when we know we've made the mistake and we pay attention to the error. Moser's study is interesting because it shows that we don't even have to be aware that we've made a mistake for brain sparks to occur. Moser concluded two interesting things. First, they found that students' brains reacted with greater ERN and Pe responses when they made mistakes than when their answers were correct. Second, they found that brain activity was greater following a mistake for students who had a growth mindset compared to those with a more fixed mindset. Those people with growth mindsets had a greater awareness of their errors were more likely to go back and correct errors. So, there was a greater chance with these people that more brain sparks were likely to occur.

All this brain research is interesting, but what can we do in our classrooms to change the way children feel about making mistakes? Historically, math has always been so focused on getting the correct answer that it is hard to change our way of thinking about wrong answers. We really need to do things in our classroom to change that message.  There's a great idea in the book about a teacher who starts math class by asking students to crumble up a piece of paper. Then students throw it at the board thinking about the feeling they had when they have made mistakes in math.  Then students get their papers back, smooth them out and trace all the crumple lines with markers. The teacher explains that the crumple lines all represent brain growth that we experience when we make mistakes. Then the students keep these papers in a math folder or notebook.
Another idea referenced in the book is something called "My favorite mistake." When students are solving problems in math, choose a wrong answer that has a lot of right things in it, but there's just one little conceptual mistake. Share that with the class and focus on all the right things in the answer. Then focus on the mistake and how students can learn from it.  There's a great video from the Learning Channel called "My Favorite No" where an middle school math teacher does something like this. Doing things like this can start to change our students' thinking about mistakes.
I hope that these two ideas are things that you might be able to use with your own students.  Stay tuned- I will share a lot more ideas and insights from this amazing book.

Number Talk Accountability

You know how it is- you are doing an amazing number talk with your students. They are sharing all sorts of interesting mental math ways to solve the problems and you think, "This is good!" Then you realize that you only have maybe half of your students (and somedays that is a high estimate) really engaged in the process. The rest, and in my case, it is usually my lower kiddos are really not engaged or paying attention to the strategies. Either they are lost because the strategies are beyond their realm of thinking at the time or they just are not engaged.  What can you really do and still maintain the structure and purpose of the number talk?
One quick fix is to prepare a set of double sided cards. You choose the colors- mine are blue and green. I used leftover cardstock and I cut 3 x 5 inch cards out of the cardstock and glued them together so I have blue on one side and green on the other. Then, I laminated them. At the start of the number talk, I give the cards to the students. I explain that they start with the cards in their laps. When they know the answer, they place the card on their desk- they can choose which side is up. If it's blue, that means they have an answer, but they don't want to share. If the card is green, they have an answer and they are willing to share their strategy. This is a quick way to make students accountable, and it makes it easy for you to choose someone to share.
Another way to make students accountable is to ask them at the end of the number talk to use finger signals to indicate the most efficient strategy. After students have shared their strategies (and you've numbered them) ask students to choose which strategy they think is most efficient. By doing this you can informally see where the students are in their thinking and can plan accordingly with additional number talks.
A third way to provide some accountability is to hold small-group number talks throughout the week. You can easily add a quick number talk to your small group math instruction. And, holding the number talk in a small group allows you to really hone in on particular skills that certain students need.
Class anchor charts explaining different strategies is another great way to hold students accountable. When you make the strategies visible in the classroom, it provides a great reference for the students and holds them accountable because the information is right there on the wall!
Another way to hold students accountable for the strategies they are learning in number talks is by asking them to solve an exit ticket problem. Give students an index card or half-sheet of paper. Pose a problem that will require them to use the strategies you've been working on. Ask students to use one side of the paper to solve the computation using a strategy that was shared during number talks. On the other side of the paper, they can solve it with their choice of strategy.  Doing this give you a quick look at individuals and their understandings and misconceptions. It also helps you choose the next direction you want to go with further number talks.  But, don't use these as a grade- simply use the information gathered to guide your instruction.